Dynamical systems theory provides powerful tools to help understand problems related to the complex climate system. The TCD group engages in the development of the theory, such as bifurcation theory, Boolean delay equations, mixing and transport, as well as their applications. Other theoretical efforts include the stability of fluid flows and the dynamics of planetary orbits.

Numerical bifurcation theory

Many systems in nature exhibit different regimes of temporal evolution pattens, depending on the values of key parameters. Bifurcation theory, combined with recent developments in numerical techniques and computational resources, enables us to determine "when and how" the transition from one regime of behavior to another occurs. Examples can be found in the following TCD research projects' web pages: low-frequency variability, atmosphere-ocean interaction, 3-D ocean circulation, and physical processes; see also the Department of Atmospheric Sciences brochure (Theoretical Climate Dynamics).


Boolean delay equations

The temporal evolution of many physical and biological systems is determined by feedback processes among the essential variables. The feedback mechanisms, in many cases, depend on a set of thresholds associated with the state variables; each feedback has a characteristic time scale.

Delay equations for Boolean-valued variables (Boolean delay equations: BDEs) are an appropriate mathematical framework for such situations. The feedback thresholds results in the discrete, on-off character of the variables, and the interaction time scales of the feedbacks are expressed as delays. Systems of BDEs have been shown to exhibit complicated and fascinating types of behavior, similar to and also different from systems of ordinary or partial differential equations. The theory of BDEs, initiated about a decade ago, is still being actively developed.
Fig: BDE
The figure above shows the evolution of solutions, x1=x1(t) and x2=x2(t), for a system of two first-order BDEs that exhibits increase of complexity in time. As time progresses, the number of "jumps" per unit time increases according to a power law.


Mixing and transport in systems with few degrees-of-freedom (d-o-f)

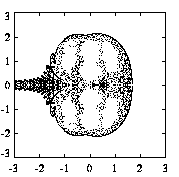Fig: dipole The applications of chaotic mixing and transport theory to the atmosphere and oceans are now being vastly expanded owing to two main reasons: 1) the development of the theory for systems with more than two d-o-f, and 2) improving computational resources and visualization techniques.



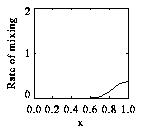
Fig: mixing rate




The figure at right shows a streak line around a pulsating dipole structure, called "thermon," in a two-layer model of oceanic flow, roughly indicating the mixing region. The figure at left shows the mixing rate between the dipoles interior and exterior regions as a function of the pulsation's amplitude.


Planetary orbits

The Earth's climate is influenced by external forcing such as variability in the Sun's radiative output and changes in the Earth's orbit and orientation in space. Solar irradiance varies on a wide range of time scales and it is far from completely understood. Orbital changes, however, are fairly well known for time spans of millions of years and they leave unmistakable signatures in records of past climates (see also Quaternary glaciation cycles). On time scales of tens of millions of years, however, the motion of planets is chaotic. While the effect of this chaos on the Earth's climate is difficult to ascertain, planetary orbits pose problems which are important and difficult in their own right.

The understanding of planetary orbits is based on Hamiltonian dynamics, averaging and perturbation theory. Due to their mutual gravitational attraction, planets perturb each other in various ways, on time scales of years and hundreds of years to thousands and millions of years. After averaging over the former, fast variations in their orbits, one obtains the so-called secular system. The dynamics of the linear part of this system can be described by its eigenmodes. One can make further simplifications by considering the phases of these modes relative to that of the largest one.

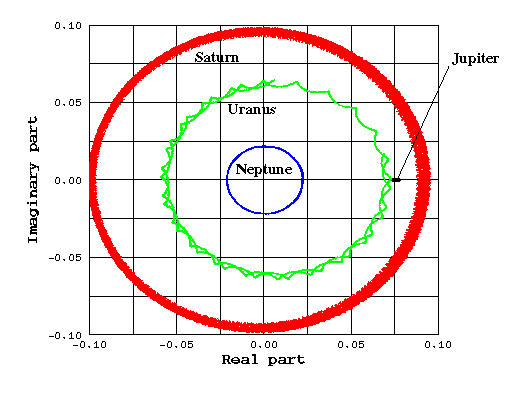
Figure: The secular motion of the Jovian planets.
The above figure depicts the long-term changes in the orbits of the Jovian planets. A transformation to the eigenmodes of the secular system was applied and then the phases were reduced relative to Jupiter's main eigenmode. The radial variable measures the amplitude of the modes, while the angle variables measure the phases relative to the phase of Jupiter's main eigenmode. Purely linear interactions would produce circles and Jupiter's main mode would appear as a point in this representation. The thickness of the curve in the case of Saturn is caused by the 2:5 orbital near-resonance between Jupiter and Saturn, the so-called Great Inequality. The variations in the case of Uranus are caused by Saturn: Saturn is the more massive of the two and it revolves around the origin at a rate faster than that of Uranus.

Our work on planetary motions includes topics such as Lie series, singularly weighted symplectic forms, the Great Inequality, secular resonances and the effects of dissipation on Hamiltonian dynamics. We also developed codes for the numerical integration of planetary orbits.


The latest results on Earth's orbital history show a particularly intriguing chaotic transition 65 million years ago which could be related to the KT impact.



The stability of fluid flows

The stability of fluid flows can be investigated by the energy-Casimir method. The original idea, due to V. I. Arnold, is to apply Lyapunov's direct method to the flow's total energy, plus a Casimir functional. In Arnold's original work, the flow under consideration needed to be a global extremum of the energy-Casimir functional. This stringent condition limits the applicability of the method and is related to the fact that the energy-Casimir functional is not sufficiently differentiable in general. One can, however, replace the energy-Casimir functional with a so-called supporting functional which is a twice Fréchet differentiable majorant of the energy-Casimir. As a result, one can relax the requirement of a global extremum and obtain stability in the case of local extrema and saddle points.

TCD Members:
M. Ghil, K. Ide, A. Saunders, F. Varadi
References:
Dee, D., and M. Ghil, l984: Boolean difference equations, I: Formulation and dynamic behavior, SIAM J. Appl. Math., 44, lll-l26.
Ghil, M., and A. Mullhaupt, l985: Boolean delay equations: periodic and aperiodic solutions, J. Stat. Phys., 41, l25-l74.
Ghil, M., and G. Wolansky, 1992: Non-Hamiltonian perturbations of integrable systems and resonance trapping, SIAM J. Appl. Math., 52, 1148-1171.
Ghil, M., F. Varadi, and W. M. Kaula, 1996: On the secular motion of the Jovian planets, Dynamics, Ephemerides and Astronomy in the Solar System, S. Ferraz-Mello, B. Morando and J. E. Arlot (Eds.), IAU Symp. No. 172, pp. 57-60.
Ide, K., and M. Ghil, 1995: An analytical study of geophysical flow estimation and mixing using extended Kalman filtering for vortex models. In Proc. 2nd WMO Ont'l Symp. on Assim. of Obs. in Meteor. & Oceanogr., Tokyo, March 1995, WMO/TD-No. 651, PWPR Report Series No. 5, WMO, Geneva, Switzerland, Vol. I, pp. 159-163.
Sakuma, H., and M. Ghil, 1990: Stability of stationary barotropic modons by Lyapunov's direct method, J. Fluid Mech., 211, 393-416.
Sakuma, H., and M. Ghil, 1991: Stability of propagating modons for small-amplitude perturbations, Phys. Fluids A, 3(3), 408-414.
Varadi, F., M. Ghil, and W. M. Kaula, 1995: The great inequality in a Hamiltonian planetary theory, From Newton to Chaos, A. E. Roy and B. A. Stevens (Eds.), Plenum Press, NY, pp. 103-108.
Varadi, F., C. M. de la Barre, W. M. Kaula, and M. Ghil, 1995: Singularly weighted symplectic forms and applications to asteroid motion, Celest. Mech. Dyn. Astron., 62, 23-41.
Wolansky, G., and M. Ghil, 1995: Stability of quasi-geostrophic flow in a periodic channel, Phys. Lett. A, 202, 111-116.
Wolansky, G., and M. Ghil, 1996: An extension of Arnol'd's second stability theorem for the Euler equations, Physica D, 94, 161-167.
Wolansky, G., M. Ghil and F. Varadi, 1996: Dynamical effects of the solar nebula's density gradient on asteroids in resonance with Jupiter, Icarus, 132, 137-150.
Varadi, F., M. Ghil and W. M. Kaula, 1998: Jupiter, Saturn and the edge of chaos, Icarus, 139, 111-116.

Last modified: 2/23/03